[surface_logic/msunit/part_one]




















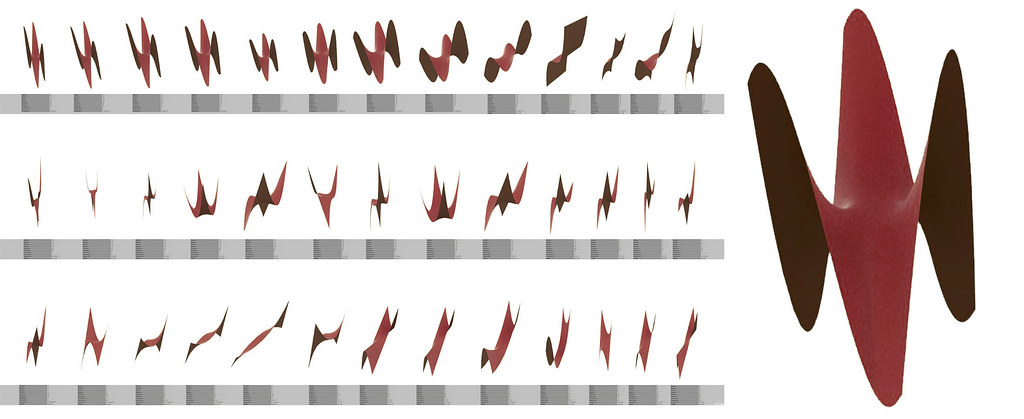
The 'Surface Logic' study originated as an exercise by which to explore mathematically derived surfaces using parametric maniplulations of differential equations. We (being Peter Beaugard in the 3D department, David Lambert of the Photo department and of course myself) are using a PlugIn for Rhino called oddly enough 'math plugin v1.0' created by Andrew Saunders and Jess Maertterer of Rensselaer Polytechnic Institute in New York. The parametric manipulations can significantly alter the outcome of the derived surface as can be seen in the 39 iterations shown above. In working through all of the above (and more) parametric iterations I was able to gain a significant amount of control of the math object's form.

This surface is known as a 'monkey saddle' as it has three depressions (one for each leg and one for the tail) so that a monkey could sit on it (if the monkey so desired). This saddle surface is described by the equation z=x^3-3xy^2.

My input into the Math Plugin Ver.1.0 to achieve the final selected surface above:
minimum u value: -1.5
maximum u value: 1.5
minimum v value: -1.5
maximum v value: 1.5
point count u: 20
point count v: 20
function x(u,v): u
function y(u.v): v
function z(u,v): u^3-1.88*u*v^2