2.17.2006
[growing_architecture/?_?_?]
can you grow a building?
can you grow a building?
2.05.2006
[erosion_table_study/test_2/results]


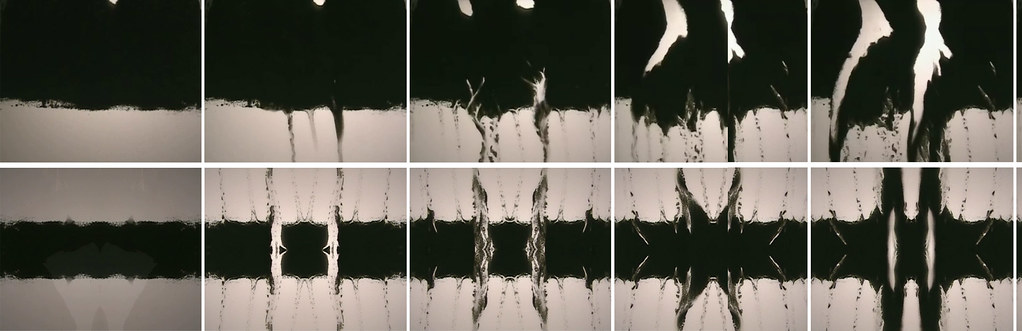
with this study i sought to establish a process that could create diversity while remaining entirely self-similar. i constructed a lighted flood table out of white acrylic that would highlight the movement of water through the black sand upon the table. i used two different water sources for these tests and the path that the water took through the material on test number three created the top series of images that you can see above. in wanting to establish a control over this natural process i forced a symmetry onto the video footage and created patterns out of the previously untouched footage. through this forced symmetry the apparent randomness of the above footage dissipated and became more natural, establishing nearly organic characteristics.


with this study i sought to establish a process that could create diversity while remaining entirely self-similar. i constructed a lighted flood table out of white acrylic that would highlight the movement of water through the black sand upon the table. i used two different water sources for these tests and the path that the water took through the material on test number three created the top series of images that you can see above. in wanting to establish a control over this natural process i forced a symmetry onto the video footage and created patterns out of the previously untouched footage. through this forced symmetry the apparent randomness of the above footage dissipated and became more natural, establishing nearly organic characteristics.
[erosion_table_study/test_3/results]
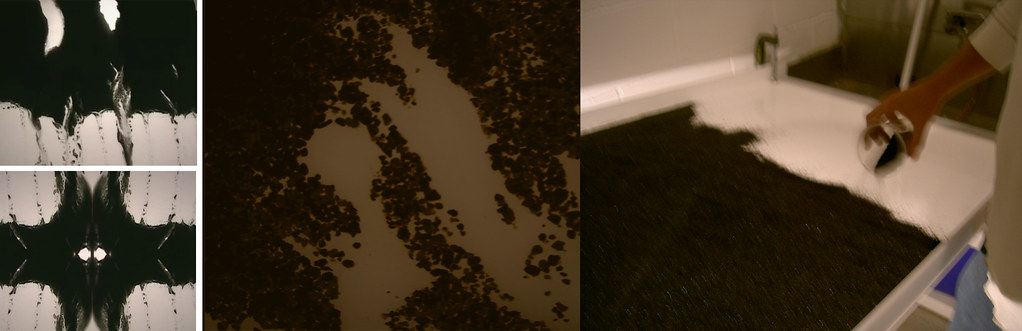

i calculated the fractal dimension of each test using the box counting method which is used primarily in calculating the fractal dimension of coastlines and other similar fractals. by imposing a square unit grid over an overhead image of the test, and then counting the number of boxes that the test’s coastline came into contact with, a resulting value was achieved. this same process was repeated as the imposed unit grid became finer, revealing a data set that is graphed onto the double logarithmic plot above. the resulting line indicates the slope for the data set revealing the calculated fractal dimension.
the fractal dimension of this particular study was 1.30074. a straight line for example has a dimension of 1 while a square has a dimension of 2. this particular test’s fractal dimension is very similar to the koch snowflake’s dimension of 1.25.



i calculated the fractal dimension of each test using the box counting method which is used primarily in calculating the fractal dimension of coastlines and other similar fractals. by imposing a square unit grid over an overhead image of the test, and then counting the number of boxes that the test’s coastline came into contact with, a resulting value was achieved. this same process was repeated as the imposed unit grid became finer, revealing a data set that is graphed onto the double logarithmic plot above. the resulting line indicates the slope for the data set revealing the calculated fractal dimension.
the fractal dimension of this particular study was 1.30074. a straight line for example has a dimension of 1 while a square has a dimension of 2. this particular test’s fractal dimension is very similar to the koch snowflake’s dimension of 1.25.